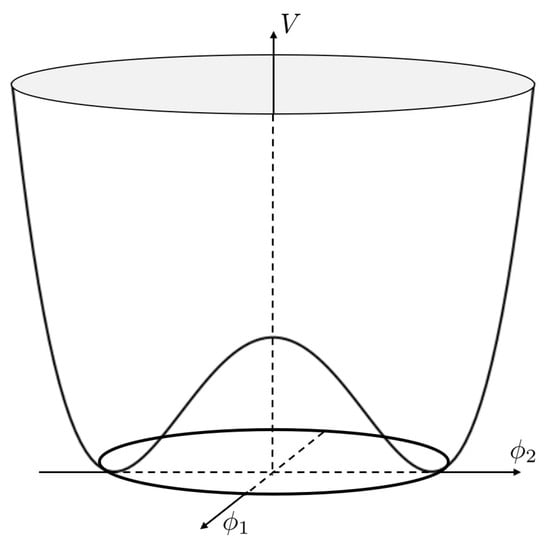
Black holes are compact objects containing huge amounts of mass in a very small spacetime region [1,2]. They develop a surface called the event horizon. Once a particle enters the event horizon, classically, it can never escape, no matter how much energy is invested in the process. Initially, it was believed that black holes were completely black, unable to emit particles. However, it was subsequently demonstrated by Hawking that the black holes also emit radiation [3,4]. This result was important because without this emission of radiation, some fundamental laws of thermodynamics would be violated [5,6,7]. For example, the first law of thermodynamics requires an appropriate definition of temperature in order to respect energy conservation. The Hawking radiation, however, brought, by itself, one problem: namely, the famous information paradox [8]. The black hole information paradox had been formulated by Hawking by the time he discovered the black hole evaporation process. Hawking realized that while the radiation emerging from a black hole is thermal in nature, and it only depends on the mass, charge, and angular momentum of the black hole, there are still an infinite number of ways to generate the same black hole with the same macroscopic properties. Thus, the same thermal radiation coming from a black hole, could be developed by any of the infinite possible microstates (internal states) consistent with the black hole macrostate with mass M, charge Q and angular momentum J. While each internal configuration of the black hole represents a pure state, the thermal radiation, being associated with all possible internal configurations, is represented by a mixed quantum state. This is the case because there is no correlation between thermal radiation, and as such, information cannot be carried out from the black hole. This lack of correlation occurs because the backreaction of the emission is not being counted. This is basically the root of the information paradox. In the past, other authors have proposed potential solutions to the black hole information problem. In [9], a possible solution was found when a non-thermal spectrum was found after calculating the backreaction. Then, in [10], it was demonstrated that there are correlations in a non-thermal spectrum. It was also found in [11] that entropy is conserved during black hole evaporation. In this paper, we take a different point of view and we propose an argument for solving the paradox. The starting point is the fact that black hole evaporation can be expressed as a natural consequence of the spontaneous breaking of the symmetry under exchange of internal configurations [12]. This symmetry keeps the same mass M, angular momentum L, and charge Q invariant. From this perspective, the vacuum expectation value of the particle number, defined as $<0|{\widehat{n}}_i|0>$, corresponds to an order parameter, being zero before the formation of the black hole and non-zero after its formation. When the black hole has some specific mass, angular momentum, and charge, it has infinite possible ground states. In other words, we have a vacuum degeneracy, typical from in processes involving spontaneous symmetry breaking. When the black hole selects one among the many possible ground states, it emits radiation, and this is equivalent to tracing out all the other possible vacuum states. The difference between this case and the ordinary breaking of spontaneous symmetry is that here, we have the possibility of having an entanglement between the different possible ground states. The information paradox disappears when we sum all the possible order parameters (particle number operators), showing that this sum must be equal to zero, and recovering, in this way, the trivial ground state before the gravitational collapse. Then, the original ground state, before the formation of the black hole, is equal to the sum of all the possible ground states after the formation of the black hole. We can interpret this as the possibility of emitting antiparticles in addition to the ordinary particles.